

- #Best explanation of geometric and arithmetic sequences how to#
- #Best explanation of geometric and arithmetic sequences series#
- #Best explanation of geometric and arithmetic sequences free#
#Best explanation of geometric and arithmetic sequences series#
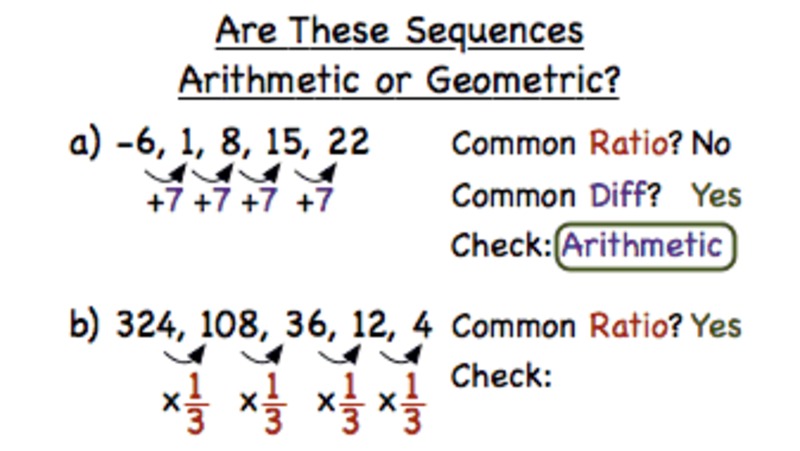
is an infinite geometric series with a = 1 and r = 1/2. Taking 's 2' as common factor, the sum of areas is, s 2 ( 1 + 1/2 + 1/4 +. The areas of squares thus formed are, s, s 2/2, s 2/4, s 2/8. If a side of the first square is "s" units, determine the sum of areas of all the squares so formed? A third square is drawn inside the second square in the same way and this process is continued indefinitely. If |r| ≥ 1, then the geometric series diverges and it cannot have a sum.Įxample: A square is drawn by joining the midpoints of the sides of the original square.If |r| Note that for any infinite geometric series, This is the formula for the sum of infinite GP. Let us assume the sum of all these infinite number of terms be S ∞. (up to an infinite number of terms) such that the absolute value of its common ratio is less than 1. But what if we have to find the sum of an infinite GP? Let us consider a GP a, ar, ar 2. The above formula gives the sum of a finite GP. We have got the same answer using the GP sum formula also. Here, the first term is, a = 2, the common ratio is, r = 2, and the number of terms is, n = 6 (as we want the sum of the amounts after 6 weeks). Clearly, this is a geometric progression as 4/2 = 8/4 = 16/2 =. The amounts saved by Clara in the order of weeks are, 2, 4, 8, 16. Now, let us work on the example (from the last section) using the sum of n terms of GP formula. What happens when r = 1? Then the GP is of form a, a, a. Subtracting equation (1) from equation (2): Let S be the sum of the geometric progression of n terms. Then the first 'n' terms of GP are of the form a, ar, ar 2.

Consider the sum of the first n terms of a geometric progression (GP) with first term a and common ratio r.
#Best explanation of geometric and arithmetic sequences how to#
It may be worth remembering that if should go offline for whatever reason, there is a mirror site at that contains most of the resources that are available here on 's discuss how to calculate the sum of n terms of GP. The short URL, ready to be copied and pasted, is as follows:Īlternatively, if you use Google Classroom, all you have to do is click on the green icon below in order to add this activity to one of your classes. If you found this activity useful don't forget to record it in your scheme of work or learning management system. NavigateĮxercises, puzzles and Maths lesson starters grouped by topic. The topic you are studying at school at the moment perhaps. Maths MapĪre you looking for something specific? An exercise to supplement
#Best explanation of geometric and arithmetic sequences free#
Page is an alphabetical list of free activities designed for One way toĪddress the problem is through the use of interactive activities and Traditional teaching fails to actively involve students. Learning and understanding Mathematics, at every level, requires Lesson Finishers then sign up for a subscription now: Newsletter, unlock the printable worksheets and see our Maths To the thousands of Transum resources, receive our monthly If you would like to enjoy ad-free access Have access to reports of the Transum Trophies earned by class Plans and assessment data in the Class Admin application and Subscribers can manage class lists, lesson Transum Topic pages and the facility to add to the collection The teacher with access to quality external links on each of the To the online exercises, quizzes and puzzles. Logged in to their Transum subscription on this computer.Ī Transum subscription unlocks the answers They are available in this space to teachers, tutors and parents You can read the newsletter online or listen to it by downloading the podcast. Click on the image above to read about the latest developments on this site and try to solve the puzzle of the month. The Transum Newsletter for October 2023 has just been published. Transum breaking news is available on Twitter and if that's not enough there is also a Transum Facebook page. You can listen to the podcast while you are commuting, exercising or relaxing. The newsletter is then duplicated as a podcast which is available on the major delivery networks. "I have used your starters for 3 years now and would not have a lesson without one! Fantastic way to engage the pupils at the start of a lesson."Įach month a newsletter is published containing details of the new additions to the Transum website and a new puzzle of the month. Thank you!"Ĭomment recorded on the 16 March 'Starter of the Day' page by Mrs A Milton, Ysgol Ardudwy: It is particularly useful when things can be saved for further use. Only recently been discovered but is used daily with all my classes. AreĬomment recorded on the 28 May 'Starter of the Day' page by L Smith, Colwyn Bay: The people who enjoy how mystifying, puzzling and hard it is. Mathematicians are not the people who find Maths easy they are


 0 kommentar(er)
0 kommentar(er)
